Reading on: Eudoxus' concentric sphere universe 408-355 BC [360 words]
Eudoxus was a Greek philosopher, astronomer, and mathematician who accepted Plato's notion of the rotation of the planets around the Earth on crystalline spheres, but noticed discrepancies with observations. He tried to adjust Plato's model by postulating that each crystalline sphere had its poles set to the next sphere. His model contained no mechanical explanation; it was simply a mathematical description but it formed the basis for the theory of concentric spheres to account for the motion of the planets.
Eudoxus calculated the length of the year as 365 days and 6 hours.
Unfortunately none of Eudoxus' writings have survived to the present-day, and we have only the accounts of others to go on. Aristotle, a contemporary of Eudoxus devotes a passage in his Metaphysics to the Eudoxan spheres.
The following excerpt is taken from Chapter 8 Book XII of Aristotle's Metaphysics translation by W. D. Ross
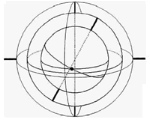
Eudoxus supposed that the motion of the sun or of the moon involves, in either case, three spheres, of which the first is the sphere of the fixed stars, and the second moves in the circle which runs along the middle of the zodiac, and the third in the circle which is inclined across the breadth of the zodiac; but the circle in which the moon moves is inclined at a greater angle than that in which the sun moves.
And the motion of the planets involves, in each case, four spheres, and of these also the first and second are the same as the first two mentioned above (for the sphere of the fixed stars is that which moves all the other spheres, and that which is placed beneath this and has its movement in the circle which bisects the zodiac is common to all), but the poles of the third sphere of each planet are in the circle which bisects the zodiac, and the motion of the fourth sphere is in the circle which is inclined at an angle to the equator of the third sphere; and the poles of the third sphere are different for each of the other planets, but those of Venus and Mercury are the same.